In a Charles Schultz Peanuts comic Schroeder takes time off from his piano practice to teach Snoopy a bit of music theory.
Standing in front of a blackboard, Schroeder gestures with a pointer to a music symbol and says, “This is a Treble Clef.”
He shifts his pointer to another symbol and states, “This is an ampersand."
“They’re quite similar,” he continues. Then in a hushed tone he explains, “Actually ... they hate each other.”
A rivalry amongst symbols? Humorous indeed, but not entirely a laughing matter.
Such contention exists at the very foundation of music theory, and at the core of music notation. It’s the friction between A and C. It's the type of dissonance rarely discussed in
music. It's the ceaseless, grinding rub that chaffs the minds of new music students. It's the disharmony that sorely complicates the lives of music teachers who must explain the
inscrutable peculiarities of music's language. It's the perpetual vying between A and C for recognition as “Alpha Musicus.” It's the fact that over the course of recent centuries
the musical world somehow centered itself around C.
In the various walks of life the letters A and C coexist peacefully. For one simple reason. A is the accepted and logical starting point. So when ordering alphabetically A always goes first,
followed by B and then C, and so on.
The letter A has significant clout in this matter. Over millennia humankind has alphabetized trillions of objects: chapters, sections, books, charts, indexes, lists, streets, rows, seats and isles. We have centuries
of alphabetical precedence, and ordering always starts with A and you go from there. With deference C follows A by two of notches, ticks, rows, columns, tabs, sections, parts, exhibits, hierarchies,
folders, volumes, ... no one argues with that. It's the way things are.
The matter of field is practically irrelevant: A and C know their place in science, biology, business, architecture; their sequence is a universally accepted convention throughout literature
and western languages.
The sole exception is the field of music, where C somehow managed to pull something over on A ... and stole the throne. This begs the questions, "How did this come
to be? And why?"
Explaining C’s alpha status in music would require a review of countless historical events, decisions, woeful happenstances, linguistic evolutions and wranglings that continue to haunt ... like a forgotten mid-air boomerang. Such a discussion is outside the scope of this article.
Historical causes aside, the facts are clear: the letter C and the C scale somehow attained
the status of “Alpha Musicus.”
In music, A has very little going for it. Really nothing much to write home about:
- There's the A 440 tuning standard, but even this "standard" bears a checkered past. The pitch of "middle A" has ranged from 400 to 480 cycles per
second—that's quite a latitude, and A cannot gloat about such a lack of authority or certainty!
- In many countries orchestras presently tune to A 440, if not, they tune to an A near 440Hz. For many decades the standard in Europe has been A 444.
- It's much easier to find an A 440 tuning fork than a C fork.
Small consolation for A, these crumbs. In stark contrast, we find plentiful ways that musicians accept and revere C as the predominant and preeminent letter in music.
Throughout musical pedantry—in the halls of scholarly thinking—C holds forth sovereignly in the prose of music pedagogy, and rules unchallenged
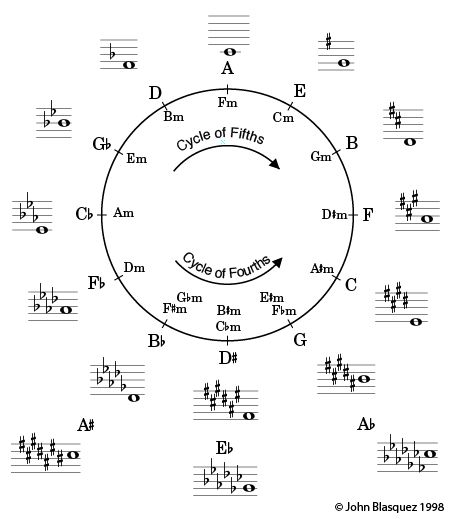
within the thick tomes of theory. C is the de facto “Do” of solfeggio. And she sits crowned and enthroned atop the Cycle of Fifths, reigning above all keys, as shown in this image of the Cycle.
The traditional Cycle of 5ths

Indeed, there is no argument. C is revered as central by those musically schooled and indoctrinated. The situation raises many questions.
Is this madness acceptable? Shouldn't the musical alphabet start at A? Why should C be the default Do? Why should C be the key with no sharps or flats?
How could a letter of lower
credential mount a credible challenge and a successful coup over A? Who let this happen? Who encouraged, designed ... or initially approved? Is there any provenance to support the outcome?
Shall we publish a list of the culprits, post it
for all to see, and demand a day for them in stocks in the public square? Shall we pejoratively refer those who whole-swallowed the C-centric view of the musical universe as spineless C-creatures?
And finally, is there anything we can do about this situation? (Is there any point in pursuing rectification? Would such an endeavor prove as futile as Dvorak keyboards vs. QWERTY, BetaMax
vs. VHS, Esperanto vs. all other languages?)
Before proposing any tonality shaking changes, let’s clearly examine the prevailing C-centric view of music.
There exist five undeniable and unjustifiable conventions that give C the upper hand over A.
1) C is the simplest scale to notate and to read. For arbitrary (well, unexplained) historical reasons, the C major scale has no sharps or flats. Only the C scale is pure, unblemished, unfettered and self sufficient, without need of chromatics (sharps or flats) in its key signature.* This single fact that bears enormous weight in C’s sway over A.
C, the one-and-only unrumpled scale, provides the "standard candle" for defining all other musical structures in field of music theory ... but in short order, because of another huge oddity — that music assigns only seven symbols to identify twelve objects, thereby created a uneven numbering system — music theory tangles terribly, nearly strangling itself by its own gangly throat.
2) C is typically the simplest scale to play. And most instruments it's readily played with uncomplicated notation and fingering. This fact is most evident on
piano. Start on a C note, then proceed up the white keys to the next C. It's like "do re mi for free." The major scale gets no simpler than that—it's something a three year old can readily comprehend and accomplish. Atop
this physical simplicity
we must appreciate the C scale’s
notational simplicity. In tandem, given the physical and notational simplicity, we have have the compelling reasons why piano instructors teach their students the C major scale before all others. (Lessons on the A scale come a considerable stretch further down the road,
often after, G, F, D, and Bb!)
Even for a transposing instrument, like an Eb tenor saxophone, the simplest major scale fingering is notated as a C scale, though it produces the "concert" sound of Eb.
3) C is usually considered the initial letter of the music alphabet. When teachers explain Do, Re, mi, their alphabetical examples use the C scale: C D E F G A B … not A B C# D E F# G#. Again this is because the C scale has no sharps or flats. As mentioned, the piano bears the closest natural relationship to music theory, and thus the C scale and the piano keyboard are the most easily depicted backbone of music theory.
4) Octave changes occur at C, not A. There are many types of "octave numbering" systems for indicating octave designations of pitches: the Helmholtz Pitch Notation System, the Alternate Helmholtz System (the Scientific pitch notation ...) or the MIDI Standard provide the simplest approach. (In Scientific pitch notation Middle C is written C4. The A below middle C in notated A3. This is because octaves are not numbered from A to A, but rather from C to C. A 440 is notated A4 — it is six notes above C4 or middle C. That of course assumes I've got my middle C octave numbering correct. Don't hold me to it.
5) C sits enthroned atop the Cycle of Fifths as previously mentioned, and shown above. The Cycle of Fifths is music theory’s organizational magnum opus. It is music’s main theoretical
and relational chart. And it's centered around C! All other keys assemble courtside around her. The key of A begrudgingly sitting three entire notches to the right of C, as if a distant heir to the throne.
Side note: The Cycle of Fifths is often referred to as the Cycle of Fourths. This name is especially logical in Jazz, where chords often progress in 4ths toward the tonic.
A-trocity
The musical deference to C bucks alphabetical traditions and customs that we implicitly accept; thus it makes no alphalogical sense. It tosses aside normal ordering conventions.
It well befuddles those newly acquainted with music. It’s tantamount to the number 3 being the first number in math and numeric counting ... or saying that we always count the seven notes
of a scale starting from 3: 3, 4, 5, 6, 7, 1, 2.
If musicians so fully honor C as the center of music, then perhaps the world’s greatest music halls should pay homage by labeling their
seating isles starting at C. Such a tribute would be small but fitting homage! Though it might confuse concert goers as much a C's prowess confuses young and budding musicians. And then ushers would be as busy as music theorists, always explaining and guiding through a situation that's permanently off kilter.
It’s a wonder that A doesn't just pack up and walk off the set. No doubt, skulking and plotting like Richard III, fueled by a feeling of entitlement and envy, A obsessively covets a throne seat atop of the Cycle of Fifths, lusting to revel amidst the due musical glory t'would follow. As with Richard, we often sympathize with the underdog, wishing, “If there only was a way.” But alas ...
In the following articles I’ll illustrate how A could successfully mount a coup ... or if nothing else, easily attain detente and reconciliation. Each article shows a quick and viable
solution for coronating A and instating her to her rightful position at the top of the Cycle of 5ths. The various solutions are so simple, I’d be surprised if no one’s previously
proposed them.
To support and clarify, I’ll present a new Cycle of Fifths for each solution, like the A-based Cycle of 5ths shown here.
An illustration of an
A-based Cycle of Fifths
Shocking, this cycle—certainly, to anyone who embraces the norm! Please though, fearlessly pull back the veil and peer down the path less taken. See how an A-based Cycle of 5ths is possible
... and why it makes sense! And that it would hardly be disruptive!
You can sit with that “clef hanger" or check for solutions in the following articles:
Solution 1 | Solution 2 | Solution 3
Author's closing note: Like the English language, music is needlessly complicated, nevertheless it works fine as it is. This series of articles was written for fun, to provide a healthy perspective shift, to help you
imagine what might have been, and what could be, and indeed what would make more sense. And to make you feel less alone in that distant gnawing feeling that all is not right.
Music theory is usually terrible dry. With rare exception it is poorly presented, and full of unsubstantiated self-substantiations. Authors frequently introduce terms before they defining them. Circular definitions abound. And for these reason, many of those who write the reference materials and teach music classes would be quickly drummed out of the engineering or mathematics departments!
The insular world of music theory deserves a good poke now and then. Satisfying to provoke thought by pointing out oddities, conventions, and by raising possibilities. And hopefully,
by taking this jaunt into music fiction, you've restimulated what you know about actual music theory.
I have no real intention of inspiring a musical insurrection. That would prove as difficult as trying to get the United States to drop the British Weights and Measures and
adopt the metric system ... now, if I were to suggest any real change ... well, how about that getting on with metric system? I was hoping that would be a plank in Obama's election platform, but that bold move would have ruined him.
More music fiction
I must admit, now I want to finish a companion article that shows how music notation would be much simpler with
a revised grand staff—additional revolutionary thoughts that again bring A to the middle!

* If you say the letters names of the major scale starting with A, you have to include a few sharps: A B C# D E F# G#. When you start from C, it goes C D E F G A B C. On most instruments
the A scale is not only harder to play, it’s harder to notate, visualize and remember.
** Stringed instruments such as violin pose a glaring exception. On violin the A scale is clearly the simplest single octave major scale. And yet, over the centuries, even violin instructors
traditionally insisted that their students first master the C scale (probably due to it’s notational purity) without regard for a few essential ergonomic matters. On violin:
- there is no C string, so there’s no open C note to provide a solid starting point
- even the simplest first position C scale spans three strings, where as the A scale started on
the A string spans two strings, and the fingering on each string is the same
- when played across all four strings the C scale requires three unique fingerings.
The A scale is clearly the simplest single octave major scale for violin!
It starts on an open string; a trip up Do Re Mi involves only two strings; doing so requires only one finger shape: whole-step whole-step half-step. Thus we have another compelling
reason not to be stridently C-centric.
TAgs / Links on this page: Traditional Cycle of 5ths